Chapter 3, Chapter 4

• SPSS

• Table 3.1 data file. for SPSS, excel format, see the below.
  • Explanation: Read the textbook for yourself (Chapter 3)
• frequency distribution
• histogram
• stem and leaf display.
  • watch How to create a Stem and Leaf Plot in Microsoft Excel
  • watch Spss

• Central Tendency (집중경향)
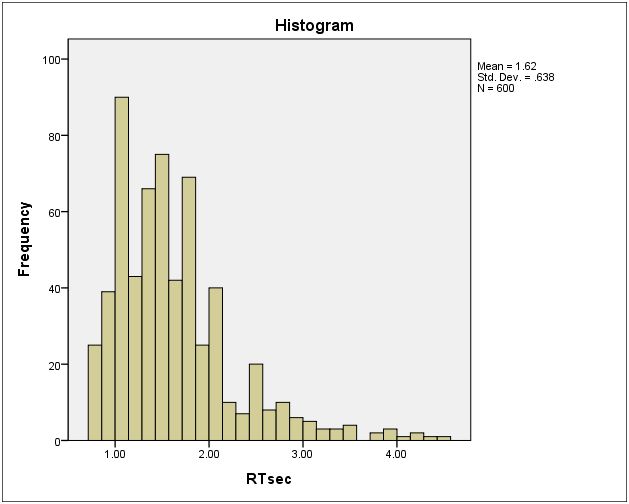
  • data: SPSS data file, rtsec or Excel file

Statistics		
RTsec 
N	Valid	600
	Missing	0
Mean		1.6245
Median		1.5300
Mode		1.33

Descriptives				
				StatisticStd. Error
RTsec	Mean			1.6245	.02603
	95% Confidence	Lower 	1.5734	
	Interval	Upper 	1.6756	
        for Mean
	5% Trimmed Mean		1.5672	
	Median			1.5300	
	Variance		.407	
	Std. Deviation		.63772	
	Minimum			.72	
	Maximum			4.44	
	Range			3.72	
	Interquartile Range	.77	
	Skewness		1.465	.100
	Kurtosis		2.849	.199

</WRAP>
<WRAP half column>
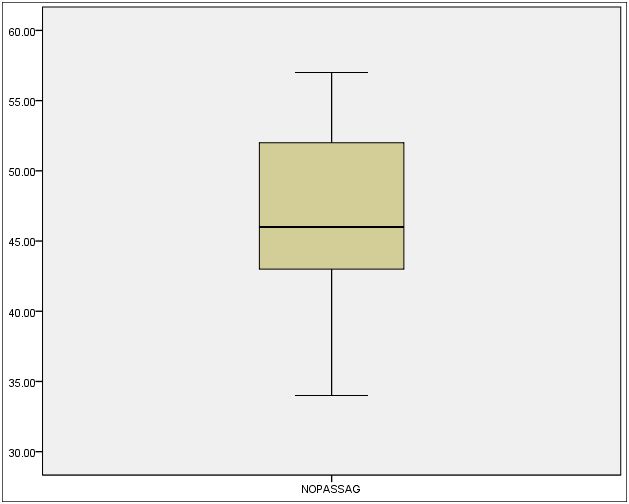
data file: ex3-1.sav 읽지 않은 지문에 대한 답을 한 학생들의 점수 (Katz, 1990).

NOPASSAG Stem-and-Leaf Plot

 Frequency    Stem &  Leaf

     1.00        3 .  4
     5.00        3 .  66689
     5.00        4 .  33444
     7.00        4 .  6666799
     5.00        5 .  01224
     5.00        5 .  55577

 Stem width:   10.00
 Each leaf:       1 case(s)

Chapter 5

• Dispersion (variability) – 분산(변산성)
• Data file: Web site or tab5-1.sav p.86-7
• range
• outlier
• 평균편차
• Variance 변량
  • 표본변량 $ s^2 $
  • 모집단변량(전집) $ \sigma^2 $

Descriptives					
	SET			Statistic	Std. Error
ATTRACT	 4	Mean		2.6445	.14651
		95% Confidence Interval for Mean	Lower Bound	2.3379	
			Upper Bound	2.9511	
		5% Trimmed Mean		2.6483	
		Median		2.5950	
		Variance		.429	
		Std. Deviation		.65520	
		Minimum		1.20	
		Maximum		4.02	
		Range		2.82	
		Interquartile Range		.82	
		Skewness		-.001	.512
		Kurtosis		.438	.992
	32	Mean		3.2615	.01541
		95% Confidence Interval for Mean	Lower Bound	3.2292	
			Upper Bound	3.2938	
		5% Trimmed Mean		3.2622	
		Median		3.2650	
		Variance		.005	
		Std. Deviation		.06892	
		Minimum		3.13	
		Maximum		3.38	
		Range		.25	
		Interquartile Range		.11	
		Skewness		-.075	.512
		Kurtosis		-.863	.992

• Standard Deviation 표준편차

• $\displaystyle S_x^2 = \displaystyle \frac {\Sigma X^2 - \frac{(\Sigma X)^2}{N} } {N-1} $

• $\displaystyle \sigma_x^2 = \displaystyle \frac {\Sigma X^2 - \frac{(\Sigma X)^2}{N} } {N} = \displaystyle \frac {\Sigma X^2}{N} - \frac {(\Sigma X)^2}{N^2} = \displaystyle \frac {\Sigma X^2}{N} - \bigg(\frac {\Sigma X}{N}\bigg)^2 = \displaystyle \frac {\Sigma X^2}{N} - \mu^2 $

• Degrees of Freedom N-1
  • Why n-1

• Sampling Distribution
• Central Limit Theorem
• Standard Error